Making a lever

[SmartAlecLights]

Hi all.
I know the principal of how it works in my head,
I just cant remember the math to work it out.
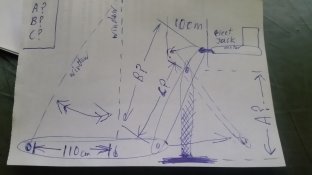
Basicly im wanting to open a window 110cm, with a 10cm electric bed (screw/piston) motor (rated at 220kg)
I also see this idea being used at Halloween to make small props big etc.
Any Formula's on how i would work out the Values for A,B,C ? would be great (correct answers would be even better)

 

[BooY]

Posted in Chat but incase you didn't see it, Its been ages since calc'ing this stuff, but I think this is what you need
A: = 550mm ish ---- Terry is Correct I forgot about the Radius on this one, its 550sih straight line from the pivot point, to cover arc needs to be larger than 778
C: = 778mm ish (Long Side)
= 70-71mm ish (Short Side)
B: = 850mm ish

View: https://drive.google.com/file/d/126PbW7CuwhxmBrAKBIA6ZBJoEaBwpr8d/view?usp=sharing


If you are looking for a smaller footprint maybe look at a Sissor action arangement, look up Caravan Sissor Stairs for some simple examples.

View: https://drive.google.com/file/d/12AoJAlzzbokAbpKLniXNNj3FUJDxIu3c/view?usp=sharing

 

[TerryK]

As @BooY indicated, the mechanical advantage of a lever is the ratio between their arm lengths. Mechanical advantage is also the ratio between the lever forces, but here it is distances. As you have it the ratio needs to be 11. That's the 110 CM for the windows divided by the 10 cm actuator. Or, C / (B - C).
The arm ratio cannot be any less than 11 as then the window would not move the necessary distance. The ratio could be greater than 11 but then the actuator distance would need to be restricted.
Something to keep in mind is that levers multiply either force or distance. Never both at the same time and if a lever multiplies force then distance is a factor of division and vice versa. So if the actuator is rated at 220 Kg the the force required to move the window must be 22 20 Kg or less. A factor of 11 less.
Edit (2022Feb05): Due to the non-perpendicular angle between the actuator and lever arm at the end of travel (either end), the actuator force transferred to the arm is a vector of the angle between the 2 which means less than the 220 Kg transferred. Only when the actuator angle relative to the lever arm is equal to 90 degrees is the full 220 Kg transferred to the lever. Also with the arm ratio of 11, 20 Kg, not 22 Kg as pre-edit indicated.

I am not sure I understand "A" as that appears to be a stand. If so then "A" needs to be longer than "C" by the radius of the pins at the both ends plus any additional material past the pin making the material strong enough for the pins not to break through the arms ends.

Couple other thoughts: worst case scenario for the arm length from the fulcrum to the actuator is 5 cm. That length would require the arm to rotate 180 degrees aside from a few other mechanical issues best avoided. The actuator arm maximum length likely will become a factor of 11 limit via the window arm. As the lever arm is sweeping an arc, both the actuator and the window 'drawbar' non-lever ends will need to be able to rotate. Your drawing shows that on the window 'drawbar' but not the actuator.